5 Soliton existence domain

We next investigate the conditions for existence of solitons. First, we need to ensure that the origin at $\phi=0$ is a root and a local maximum of $\Psi$ in Eq. (33), i.e., $\Psi(\phi)=0$, $\Psi^{\prime}(\phi)=0$ and $\Psi^{\prime\prime}(\phi)<0$ at $\phi=0$ [43,44,45], where primes denote derivatives with respect to $\phi$. It is easily seen that the first two constraints are satisfied. We thus impose the condition

$$F_{1}(M)=-\left. \Psi^{\prime\prime}(\phi)\right\vert _{\phi=0}=\frac {\beta(\kappa-\frac{1}{2})}{\kappa-\tfrac{3}{2}}-\frac{1}{M^{2}-3{\sigma}}>0.$$ (34)

Eq. (34) provides the minimum value for the Mach number, $M_{1}$, i.e. :

$$M>M_{1}=\left[ \frac{\kappa-\tfrac{3}{2}}{\beta(\kappa-\frac{1}{2})} +3{\sigma}\right] ^{1/2}.$$ (35)

Clearly, $M_{1}$ is the (normalized) electron-acoustic phase speed - cf. Eq. (16). It is thus also related to Debye screening via the screening parameter $\lambda_{D,\kappa}$ in (15), associated with the hot $\kappa$-distributed electrons. We deduce that soliton solutions are super-acoustic. For Maxwellian hot electrons ( $\kappa\rightarrow\infty$) and cold ``cool'' electrons ($\sigma=0$), we obtain $M_{1}=1/\beta^{1/2}$, thus recovering the normalized phase speed for electron-acoustic waves in a Maxwellian plasma. [5] The lower Mach number limit, $M_{1}$, increases with $T_{c}$ (via $\sigma$), and decreases for lower values of $\kappa$ (large excess of suprathermal electrons), and hence the sound speed in suprathermal plasmas is reduced, in comparison with Maxwellian plasmas ( $\kappa\rightarrow\infty$).

An upper limit for $M$ is found through the fact that the cool electron density becomes complex at $\phi=\phi_{max}$, and hence the largest soliton amplitude satisfies $F_{2}(M)=\Psi(\phi)\vert _{\phi=\phi_{\max}}>0$. This yields the following equation for the upper limit in M:

$$F_{2}(M) =-\frac{1}{2}(1+\beta)\left( {M-}\sqrt{3{\sigma}}\right) ^{2}-\frac{4}{3}M^{3/2}\left( 3{\sigma}\right) ^{1/4}$$

$$+\beta\left( 1-\left[ 1+\frac{\left( {M-}\sqrt{3{\sigma}}\right) ^{2} }{2\kappa-3}\right] ^{-\kappa+3/2}\right)$$

$$+M{{^{2}+}\sigma} =0\,.$$ (36)

Solving Eq. (36) provides the upper limit $M_{2}(\kappa,\beta ,\sigma)$ for acceptable values of the Mach number for solitons to exist.

For comparison, for a Maxwellian distribution (here recovered as $\kappa\rightarrow\infty$), the constraints reduce to

$$F_{1}(M) =\beta-\frac{1}{M^{2}-3{\sigma}}>0,$$ (37)

$$F_{2}(M) =-\frac{1}{2}(1+\beta)\left( {M-}\sqrt{3{\sigma}}\right) ^{2}-\frac{4}{3}M^{3/2}\left( 3{\sigma}\right) ^{1/4}$$

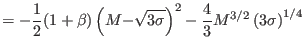$$+\beta\left( 1-\exp\left[ -\tfrac{1}{2}\left( {M-}\sqrt{3{\sigma} }\right) ^{2}\right] \right)$$

$$+M{{^{2}+}\sigma}>0.$$ (38)

The latter equation provides the upper limit $M_{2}$, while the lower limit becomes $M_{1}=(1/\beta+3\sigma)^{1/2}$.

In the opposite limit of ultrastrong suprathermality, i.e., $\kappa \rightarrow3/2$, the Mach number threshold approaches a non-zero limit $M_{1}=\sqrt{3{\sigma}}$, which is essentially the thermal speed, as noted above (recall that $M>\sqrt{3{\sigma}}$ by assumption). The upper limit $M_{2}$ is then given by

$$F_{2}(M) =-\frac{1}{2}(1+\beta)\left( {M-}\sqrt{3{\sigma}}\right) ^{2}+M{{^{2}+}\sigma}$$

$$-\frac{4}{3}M^{3/2}\left( 3{\sigma}\right) ^{1/4}=0\,.$$ (39)

Interestingly, the two limits $M_{1}$ and $M_{2}$ both tend to the same limit as $\kappa \rightarrow3/2$, namely, $\sqrt{3{\sigma}}$, where the soliton existence region vanishes, as the kappa distribution breaks down.

We have studied the existence domain of electron-acoustic solitary waves for different values of the parameters. The results are depicted in Figs. 2-3. Solitary structures of the electrostatic potential may occur in the range $M_{1}<M<M_{2}$, which depends on the parameters $\beta$, $\kappa$, and $\sigma$. We recall that we have also assumed that cool electrons are supersonic (in the sense $M>\sqrt{3{\sigma}}$) [43,44,45], and the hot electrons subsonic ($M<1$), and care must be taken not to go beyond the limits of the plasma model.

Figure 4: (Color online) The pseudopotential $\Psi (\phi )$ (upper panel) and the associated solution (electric potential pulse) $\phi$ (lower panel) are depicted versus position $\xi$, for different values of the temperature ratio $\sigma\,$. We have taken: $\sigma =0.01$ (solid curve), $0.02$ (dashed curve), and $0.04$ (dot-dashed curve). The other parameter values are $\beta =1.3$, $\kappa =2.5$ and $M=0.75$.

The interval $[M_{1},M_{2}]$ where solitons may exist is depicted in Fig. 2, in two opposite cases: in (a) and (c) two very low, and in (b) one very high value of $\kappa$. We thus see that for both a quasi-Maxwellian distribution and one with a large excess suprathermal component of hot electrons, both $M_{1}$ and $M_{2}$ decrease with an increase in the relative density parameter $\beta$ for fixed $\kappa$ and soliton speed $M$. Further, the upper limit falls off more rapidly, and thus the existence domain in Mach number becomes narrower for higher values of the hot-to-cool electron density ratio. Comparing the two frames (a) and (b) in Fig. 2, we immediately notice that suprathermality (low $\kappa$) results in solitons propagating at lower Mach number values, a trend which is also seen in Fig. 2c. Another trend that is visible in Figs. 2-3a is that increased thermal pressure effects of the cool electrons, manifested through increasing $\sigma$, also lead to a narrowing of the Mach number range that can support solitons. Finally, we note that for $\beta\sim1$, the upper limit found from Eq. (36) rises above the limit $M=1$ required by the assumptions of the model, and the latter then forms the upper limit.

Interestingly, in Figs. 2-3 the existence region appears to shrink down to nil, as the curves approach each other for high $\beta$ values. This is particularly visible in Fig. 2c, for a very low value of $\kappa$ ( $\kappa =1.65$). This is not an unexpected result, as high values of $\beta$ are equivalent to a reduction in cool electron relative density, which leads to our model breaking down if the inertial electrons vanish. We recall that a value $\beta>4$ is a rather abstract case, as it corresponds to a forbidden regime, since Landau damping will prevent electron-acoustic oscillations from propagating. Similarly, a high value of the temperature ratio, such as $\sigma=0.2$, takes us outside the physically reasonable domain. Nevertheless, as it appears that the lower and upper limits in $M$ approach each other asymptotically for high values of $\beta$, we have carried out calculations for increasing $\beta$, up to $\beta=100$ for $\sigma=0.2$ as an academic exercise, and can confirm that the two limits do not actually intersect.

Figure 5: (Color online) (a) The pseudopotential $\Psi (\phi )$ and the associated solutions: (b) electric potential pulse $\phi$, (c) density $n$, and (d) velocity $u$ are depicted versus position $\xi$, for different $\kappa$. We have taken: $\kappa =2.5$ (solid curve), $3$ (dashed curve), and $3.5$ (dot-dashed curve). The other parameter values are: $\sigma =0.02$, $\beta =1.6$, and $M=0.75$.

Figure 3 shows the range of allowed Mach numbers as a function of $\kappa$, for various values of the temperature ratio $\sigma$. As discussed above, increasing $\kappa$ towards a Maxwellian distribution ( $\kappa\rightarrow\infty$) broadens the Mach number range and yields higher values of Mach number. On the other hand, both upper and lower limits decrease as the limiting value $\kappa \rightarrow3/2$ is approached. The qualitative conclusion is analogous to the trend in Fig. 2: stronger excess suprathermality leads to solitons occurring in narrower ranges of $M$. Furthermore, as illustrated in Figs. 2 and 3a, the Mach number threshold $M_{1}$ approaches the upper limit $M_{2}$ for high values of $\sigma$ and $\beta$: both increased hot-electron density and cool-electron thermal effects shrink the permitted soliton existence region.

Figure 3b depicts the range of allowed Mach numbers as a function of $\kappa$ for various values of the density parameter $\beta$ (for a fixed indicative $\sigma$ value). We note that both curves decrease with an increase in $\beta$. Although it lies in the damped region, we have also depicted a high $\beta$ regime for comparison (solid-crosses curve).

We conclude this section with a brief comparison of our work with that of Ref. [39]. The latter did not consider existence domains at all, let alone their dependence on plasma parameters, but merely plotted some Sagdeev potentials and associated soliton potential profiles for chosen values of some of the parameters, so as to extract some trends. En passant, there is indirect mention of an upper limit in $M$, in that it is commented that as increasing values of $M$ are considered, at some stage solitary waves cease to exist. [39]

Figure 6: (Color online) The dependence of the pulse amplitude $\vert\phi _{m}\vert$ on the Mach number-to-sound-speed ratio $M/M_{1}$ is depicted, for different values of $\kappa$. From top to bottom: $\kappa =100$ (solid curve); $10$ (dashed curve); $4$ (dot-dashed curve); $3$ (crosses); $2.5$ (solid circles); $2$ (solid squares). Here, $\sigma =0.01$ and $\beta =1.3$.

Figure 7: (Color online) (Upper panel) The pseudopotential $\Psi (\phi )$ vs. $\phi$ and (lower panel) the associated electric potential pulse $\phi$ vs. $\xi$ are depicted, for different values of the hot-to-cold electron density ratio $\beta$. From top to bottom: $\beta =1.3$ (solid curve); $1.5$ (dashed curve); $1.7$ (dot-dashed curve). Here $\sigma =0.01$, $\kappa =2.5$ and $M=0.75$.