3.1 Dynamic Formulas

To provide equations governing relativistic dynamics of matter, we use the Bianchi identities

$$\nabla_{\lbrack e}R_{ab]cd}=0.$$ (35)

On substituting Eq. (29) into Eq. (35), we get the dynamic formula for the Weyl conformal curvature[3,40,41]:

$$\nabla^{d}C_{abcd}=-\nabla_{\lbrack a}(R_{b]c}-{\textstyle{\frac{... ...\lbrack a}(T_{b]c}-{\textstyle{\frac{1}{3}}}g_{b]c}T_{d} {}^{d})\equiv J_{abc}.$$ (36)

On decomposing Eq. (36) along and orthogonal to a 4-velocity vector, we obtain constraint ( 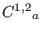$C^{1,2}{}_{a}$) and propagation ( $P^{1,2} {}_{ab}$) equations of the Weyl fields in a form analogous to the Maxwell equations[10,42,43,44]:

$$C^{1}{}_{a}\equiv {(\mathrm{div}E)_{a}}-3\omega^{b}H_{ab}-[\sigma ,H]_{a}-{\textstyle{\frac{1}{3}}}\mathrm{D}_{a}\rho+{\textstyle{\frac{1}{3}} }\Theta q_{a}$$

$$-{\textstyle{\frac{1}{2}}}\sigma_{ab}q^{b}+{\textstyle{\frac{3}{2}}} [\omega,q]_{a}+{\textstyle{\frac{1}{2}}(\mathrm{div}\pi)_{a}} =0,$$ (37)

$$C^{2}{}_{a}\equiv {(\mathrm{div}H)_{a}}+3\omega^{b}E_{ab}+[\sigma,E]_{a} +\omega_{a}(\rho+p)$$

$$+{\textstyle{\frac{1}{2}}}\mathrm{curl}(q)_{a}+{\textstyle{\frac{1}{2}} }[\sigma,\pi]_{a}-{\textstyle{\frac{1}{2}}}\omega^{b}\pi_{ab} =0,$$ (38)

$$P^{1}{}_{ab}\equiv \mathrm{curl}(H)_{ab}+2[\dot{u},H]_{\left\langle {ab}\right\rangl... ... {ab}\right\rangle }-\Theta E_{ab}+[\omega,E]_{\left\langle {ab}\right\rangle }$$

$$+3\sigma_{c\left\langle a\right. }E_{\left. b\right\rangle }{} ^{... ...t. b\right\rangle }-\dot {u}_{\left\langle a\right. }q_{\left. b\right\rangle }$$

$$-{\textstyle{\frac{1}{2}}}\dot{\pi}_{\left\langle {ab}\right\rang... ...{1}{2}}}\sigma^{e} {}_{\left\langle a\right. }\pi_{\left. b\right\rangle e} =0,$$ (39)

$$P^{2}{}_{ab}\equiv \mathrm{curl}(E)_{ab}+2[\dot{u},E]_{\left\langle {ab}\right\rangl... ... {ab}\right\rangle }+\Theta H_{ab}-[\omega,H]_{\left\langle {ab}\right\rangle }$$

$$-3\sigma_{c\left\langle a\right. }H_{\left. b\right\rangle }{} ^{... ...langle {ab}\right\rangle }-{\textstyle{\frac{1}{2}}}\mathrm{curl}(\pi)_{ab} =0.$$ (40)

The twice contracted Bianchi identities present the conservation of the total energy momentum tensor, namely

$$\nabla^{b}T_{ab}=\nabla^{b}(R_{ab}-{\textstyle{\frac{1}{2}}}g_{ab}R)=0.$$ (41)

It is split into a timelike and a spacelike momentum constraints:

$$C^{3}\equiv\dot{\rho}+(\rho+p)\Theta+{\mathrm{div}(q)}+2\dot{u}_{a} q^{a}+\sigma_{ab}\pi^{ab}=0,$$ (42)

$$C^{4}{}_{a}\equiv (\rho+p)\dot{u}_{a}+\mathrm{D}_{a}p+\dot{q}_{\left\langle a\right\rangle }+{\textstyle{\frac{4}{3}}}\Theta q_{a}+\sigma_{ab} q^{b}$$

$$-[\omega,q]_{a}+{(\mathrm{div}\pi)_{a}}+\dot{u}^{b}\pi_{ab}=0.$$ (43)

They provide the conservation law of energy-momentum, i. e., how matter determines the geometry, and describe the motion of matter.